Development of 3D flow diagnostic numerical simulator and rock property modelling for a homogeneous faulted reservoir
Keywords:
Faults, Flow diagnostics, Numerical modeling and simulation, Sweep efficiency, ReservoirAbstract
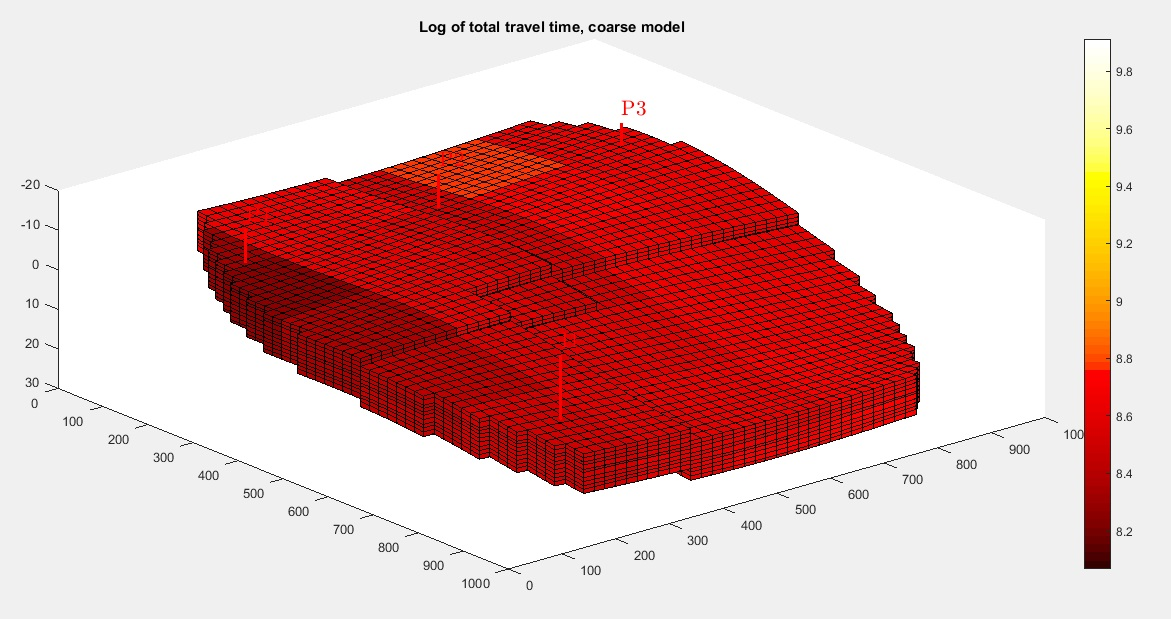
Research has shown that faults, especially sealing faults, have become a barrier to flow. Flow diagnostics, on the other hand, are straightforward and controlled numerical flow experiments that are performed to examine a reservoir model, build links and offer rudimentary volume estimates as well as to quickly produce a qualitative representation of the reservoir’s flow patterns. To evaluate, assess, and validate reservoir models and production scenarios, the ’diagnostics’ module in the Matlab Reservoir Simulation Toolbox (MRST) provides a computationally less expensive alternative to running fully featured multiphase simulations. This is done to determine qualitatively regions for well placement to maximize hydrocarbon recovery. Monitoring flow fields, timelines, and tracers—neutral particles in the fluid that flow with the fluid—are all part of flow diagnostics. These techniques are used to determine volumetric communication between a pair of injector-producer wells, as well as flow patterns between injection-production wells and the arrival time between them. To create the grid, the simulation schedule, the wells, upscaling, arrival time computation, and flow diagnostics (drainage and sweep regions) were all done using the Matlab reservoir simulation toolbox (MRST) package by “Makemodel 3” on the data obtained from “SPE 10th comparative solution project” on the MRST package. This was done for a homogeneous anisotropic faulted porous medium. The simulation runs for about 473,739 milliseconds on a fine grid having 20040 active cells while in the coarse model with faster computational time of about 200 seconds, 112 milliseconds. At the early stages of the reservoir bottom-hole pressure for injector I1(about 7000psia) and three producers P1, P2 and P3 remains constant over time (about 3600psia) thanks to pressure support by I1. The extent of residence time after 10 years for the fine, coarse and more coarser model is that fluid element find it easier to transverse through the fault line from I1 to P1, P2 then P3 which takes lesser time than crossing across two intersecting faults, It can also be seen that the sweep efficiency of the fine grid is more effective than the coarse grid. Finally, it has been seen clearly that the fine grid model gives more accurate details but slower computational time while the coarse model which is less accurate but with faster computational time.
Published
How to Cite
Issue
Section
Copyright (c) 2024 Surajo Muhammed Gwio, Ibrahim Ayuba, Umar Faruk Aminua

This work is licensed under a Creative Commons Attribution 4.0 International License.



