A Numerical Method using Collocation Approach for the Solution of Volterra-Fredholm Integro-Differential Equations

Authors

  • G. Ajileye Department of Mathematics and Statistics, Federal University Wukari, Taraba State, Nigeria
  • F. A. Aminu Department of Mathematics and Statistics, Federal University Wukari, Taraba State, Nigeria

Keywords:

Collocation, Volterra-Fredholm, Integro-di erential, Power series, Approximate solution

Abstract

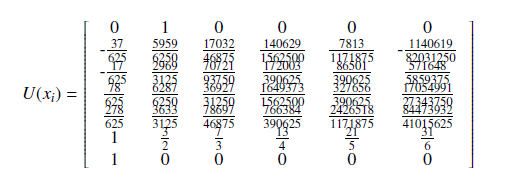
This paper consider collocation approach for the numerical solution of Volterra-Fredholm Integro-differential equation using collocation method. We transformed the problem into a system of linear algebraic equations and matrix inversion is adopted to solve the algebraic equations. We substituted the solution algebraic equations into the approximate equation to obtain the numerical result. Some numerical problems are solved to demonstrate the efficiency and consistency of the method.

Dimensions

A. M. Wazwaz & S. M. El-Sayed, “A new modification of the Adomian decompostion method for linear and nonlinear operators”, (Doctoral dissertation). App. Math. Comput. 181 (2001) 393.

D. A. Gegele, O. P. Evans & D. Akoh, “Numerical solution of higher order linear Fredholm integro-differential equations”, American Journal of Engineering Research 3 (2014) 243.

R. H. Khan & H. O. Bakodah, “Adomian decomposition method and its modification for nonlinear Abel’s integral equations”, Computers and Mathematics with Applications 7 (2013) 2349.

R. C. Mittal & R. Nigam, “Solution of fractional integro-differential equations by Adomiandecomposition method”, The International Journal of Applied Mathematics and Mechanics 2 (2008) 87.

A. O. Adesanya, Y. A.Yahaya, B. Ahmed & R. O. Onsachi, “Numerical Solution of Linear integral and Integro-Differential Equations Using Boubakar Collocation Method”, International Journal of Mathematical Analysis and Optimization: Theory and Application 2 (2019) 592.

A. O. Agbolade & T. A. Anake, “Solution of first order volterra linear integro di erential equations by collocation method”, J. Appl. Math., Article ID. 1510267 (2017). https://doi.org/10.1155/2017/1510267

S. Nemati, P. Lima & Y. Ordokhani, “Numerical method for the mixed Volterra-Fredholm integral equations using hybrid Legendre function”, Conference Application of Mathematics (2015) 184.

G. Mehdiyera, M. Imanova & V. Ibrahim, “Solving Volterra integro di erential equation by second derivative methods”, 43rd Appl. Math. Inf. Sci. 9 (2015) 2521.

G. Mehdiyeva, V. Ibrahimov & M Imanova, “On the Construction of the Multistep Methods to Solving the Initial-Value Problem for ODE and the Volterra Integro-Di erential Equations”, IAPE, Oxford, United Kingdom (2019), ISBN: 978-1-912532-05-6.

K. Issa & F. Saleh, “Approximate solution of pertubed Volterra Fredholm integro di erential equation by Chebyshev-Galerkin method”, Journal of Mathematics (2017). https://doi.org/10,1155/2017/8213932.

A. H. Bhraway, E. Tohidi & F. Soleymani, “A new Bernoulli matrix method for solving high order linear and nonlinear Fredholm integrodifferential equations with piecewise interval”, Appl. Math. Comput. 219 (2012) 482.

C. Ercan & T. Kharerah, “Solving a class of Volterra integral system by the di erential transform method”, Int. J. Nonlinear Sci. 16 (2013) 87.

M. El-kady & M. Biomy, “Efficient Legendre pseudospectral method for solving integral and integro differential equation”, Commom Nonlinear Sci. Numer Simulat (2010) 1724.

N. Irfan, S. Kumar & S. Kapoor, “Bernstein Operational Matrix Approach for Integro-Di erential Equation Arising in Control theory”, Nonlinear Engineering 3 (2014) 117-123.

S. E. Fadugba, “Solution of Fractional Order Equations in the Domain of the Mellin Transform”, Journal of the Nigerian Society of Physical Sciences 4 (2019) 138. https://doi.org/10.46481/jnsps.2019.31

Y. Nawaz, “Variational iteration method and homotopy perturbation method for fourth-order fractional integro-di erential equations”, Computers & Mathematics with Applications 8 (2011) 2330.

O. A. Uwaheren, A. F. Adebisi & O. A. Taiwo, “Perturbed Collocation Method For Solving Singular Multi-order Fractional Di erential Equations of Lane-Emden Type”, Journal of the Nigerian Society of Physical Sciences 3 (2020) 141. https://doi.org/10.46481/jnsps.2020.69.

M. K. Shahooth, R. R. Ahmed, U-K. S. Din, W. Swidan, O. K. Al-Husseini & W. K. Shahooth, “Approximation Solution to Solving Linear Volterra-Fredholm Integro-Di erential Equations of the Second Kind by Using Bernstein Polynomials Method”, J Appl Computat Math 5 (2016). https://doi.org/10.4172/2168-9679.1000298.

O. A. Uwaheren, A. F. Adebisi, O. T. Olotu, M. O. Etuk & O. J. Peter, “Legendre Galerkin Method for Solving Fractional Integro-Di erential Equations of Fredholm Type”, The Aligarh Bulletin of Mathematics 40 (2021) 15.

T.Oyedepo, O. A. Uwaheren, E. P. Okperhie & O. J. Peter, “Solution of Fractional Integro-Di erential Equation Using Modified Homotopy Perturbation Technique and Constructed Orthogonal Polynomials as Basis Functions”, Journal of Science Technology and Education 7 (2019). ISSN: 2277-0011; Journal homepage: www.atbuftejoste.com

O. A. Uwaheren, A. F. Adebisi, C. Y. Ishola, M. T. Raji, A. O. Yekeem & O. J. Peter, “Numerical Solution of Volterra Integro-differential Equation by Akbari-Ganji’s Method”, Journal of Mathematics and Its Application 16 (2022) 1123.

G. Ajileye, A. A. James, A. M. Ayinde&T. Oyedepo, “Collocation Approach for the Computational Solution Of Fredholm-Volterra Fractional Order of Integro-Di erential Equations”, J. Nig. Soc. Phys. Sci. 4 (2022) 834.

G. Ajileye & F. A. Aminu, “Approximate Solution to First-Order Integro-di erential Equations Using Polynomial Collocation Approach”, J Appl Computat Math.11 (2022) 486.

Published

2022-12-29

How to Cite

A Numerical Method using Collocation Approach for the Solution of Volterra-Fredholm Integro-Differential Equations. (2022). African Scientific Reports, 1(3), 205–211. https://doi.org/10.46481/asr.2022.1.3.58

Issue

Volume 1, Issue 3, December 2022

Section

Original Research
Copyright & Licensing

Copyright (c) 2022 G. Ajileye, F. A. Aminu

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

A Numerical Method using Collocation Approach for the Solution of Volterra-Fredholm Integro-Differential Equations. (2022). African Scientific Reports, 1(3), 205–211. https://doi.org/10.46481/asr.2022.1.3.58