Approximate solution of time-fractional non-linear parabolic equations arising in Mathematical Physics

Authors

  • K. Issa Department of Mathematics and Statistics, Kwara State University, Malete, Kwara State, P. M. B. 1530, Ilorin, Nigeria
  • R. A. Bello Department of Mathematics and Statistics, Kwara State University, Malete, Kwara State, P. M. B. 1530, Ilorin, Nigeria
  • M. H. Sulaiman Department of Mathematics and Statistics, Kwara State University, Malete, Kwara State, P. M. B. 1530, Ilorin, Nigeria

Keywords:

Iterative method, Gamma function, Parabolic equation

Abstract

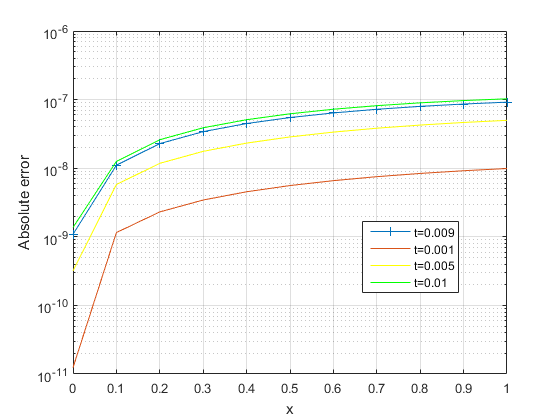
In this paper, we studied and analysed a new iterative method for solving time-fractional non-linear equations by obtaining approximate solutions to the Allen-Cahn, Newell-Whitehead, and Fisher equations by putting the parameter \alpha = 1 and varying the values of \gamma, \phi, and \tau. These three equations were derived from the general non-linear dynamical wave equations when the constants therein assumed certain specific values. Obviously, from the tabulated results, we observed that the approximate solution for each example compares favourably with the existing results in the literature; therefore, the proposed scheme is effective and accurate in solving Allen-Cahn, Newell-Whitehead, and Fisher equations. All the computational work was done using Mathematica, and all the graphs were plotted using MATLAB.

Dimensions

H. Ahmad, A. R. Seadawy, T. A. Khan & P. Thounthong, “Analytic approximate solutions for some nonlinear Parabolic dynamical wave equations”, J. Taibah. Univ. Sci. 14 (2020) 346. https://doi.org/10.1080/16583655.2020.1741943.

I. Hassan, A. O. Collins & O. I. Obiajulu, “The revised new iterative method for solving the model describing biological species living together”, Amer. J. Comp. Appl. Maths. 6 (2016) 136. https://doi.org/10.5923/j.ajcam.20160603.03.

M. N. Alam & M. M. Alam, “An analytical method for solving exact solutions of a nonlinear evolution equation describing the dynamics of ionic currents along microtubules”, J. Taibah. Univ. Sci. 11 (2017) 939. https://doi.org/10.1016/j.jtusci.2016.11.004.

H. Kheiri, N. Alipour & R. Dehghani, “Homotopy analysis and Homotopy-Pade methods for the modified Burgers-Korteweg-de-Vries and the Newell Whitehead equation”, Math. Sci. 5 (2011) 33. https://www.sid.ir/paper/322534/en.

J. Li, I. Ahmad, H. Ahmad, D. Shah, Y. Chu, P. Thounthong & M. Ayaz, “Numerical solution of two-term time fractional PDE models arising in mathematical physics using local meshless method”, Open Physics 18 (2020) 1063. https://doi.org/10.1515/phys-2020-0222.

E. Babolian & J. Saeidian, “Analytic approximate solutions to Burgers, Fishers, Huxley equations and two combined forms of these equations”, Commun. Nonlinear Sci Numer Simul. 14 (2009) 1984. https://doi.org/10.1016/j.cnsns.2008.07.019.

M. G. Sakar, F. Uludag & F. Erdogan, “Numerical solution of time-fractional nonlinear PDEs with proportional delays by homotopy perturbation method”, Applied Mathematical Modelling 40 (2016) 6639. https://doi.org/10.1016/j.apm.2016.02.005.

A. S. V. R. Kanth, K. Aruna, K. Raghavendar, H. Rezazadeh & M. Inc, “Numerical solutions of nonlinear time fractional Klein-Gordon equation via natural transform decomposition method and iterative Shehu transform method”, Journal of Ocean Engineering and Science (2021) 1. https://doi.org/10.1016/j.joes.2021.12.002.

K. Issa, B. M. Yisa & J. Biazar, “Numerical solution of space fractional diffusion equation using shifted Gegenbauer polynomials”, Computational Methods for Differential Equations 10 (2022) 431. https://doi.org/10.22034/cmde.2020.42106.1818.

K. Issa, A. S. Olorunnisola, T. O. Aliu & A. D. Adeshola, “Approximate solution of space fractional order diffusion equations by Gegenbauer collocation and compact finite difference scheme”, J. Nig. Soc. Phys. Sci. 5 (2023) 1368. https://doi.org/10.46481/jnsps.2023.1368.

G. Hariharan & K. Kannan, “Haar Wavelet method for solving some nonlinear parabolic equations”, J. Math. Chem. 48 (2010) 1044. https://doi.org/10.1007/s10910-010-9724-0.

G. Hariharan, “An efficient Legendre wavelet-based approximation method for a few Newell-Whitehead and Allen-Cahn equations”, J. Membr. Biol. 247 (2014) 371. https://doi.org/10.1007/s00232-014-9638-z.

X. Feng, H. Song, T. Tang & J. Yang, “Nonlinear stability of the implicit-explicit methods for the Allen-Cahn equation”, Inverse Problems and Imaging 7 (2013) 679. https://doi.org/10.3934/ipi.2013.7.679.

L. Akinyemi, O. S. Iyiola, & U. Akpan, “Iterative methods for solving fourth-and sixth-order time-fractional Cahn-Hillard equation”, Math. Meth. Appl. Sci. (2020) 1. https://doi.org/10.1002/mma.6173.

V. Daftardar-Gejji & H. Jafari, “An iterative method for solving nonlinear function equations”, J. Math. Anal. Appl. 316 (2006) 753. https://doi.org/10.1016/j.jmaa.2005.05.009.

S. Bhalekar & V. Daftardar-Gejji, “Solving a system of nonlinear functional equations using revised new iterative method”, Int. J. Comp. Math. Sci. 6 (2012) 1. https://doi.org/10.5281/zenodo.1329849.

M. Yaseen & M. Samrain, “The modified new iterative method for solving linear and nonlinear Klein Gordon equations”, Appl. Math. Sci. 6 (2012) 2979. https://www.m-hikari.com/ams/ams-2012/ams-57-60-2012/yaseenAMS57-60-2012.pdf.

H. Jafar, S. Ghasempour & D. Baleam, “On comparison between iterative methods for solving nonlinear optimal control problems”, Journal of Vibration and Control 22 (2016) 2281. https://doi.org/10.1177/1077546315590039.

A. Muhit & U. Amit, “A modified iterative method for solving nonlinear functional equation”, Appl. Math. Nonlinear Sci. 6 (2020) 347. https://doi.org/10.2478/amns.2020.2.00055.

M. N. Alam & X. Li, “Exact traveling wave solutions to higher order nonlinear equations”, J. Ocean Eng. Sci. 4 (2019) 276. https://doi.org/10.1016/j.joes.2019.05.003.

L. Wenjin & P. Yanni, “An Iterative Method for Time-Fractional Swift-Hohenberg Equation”, Advances in Mathematical Physics (2018) 2405432. https://doi.org/10.1155/2018/2405432.

L. G. Romero, L. L. Luque, G. A. Dorrego & R. A. Cerutti, “On the k-Riemann-Liouville Fractional Derivative”, Int. J. Contemp. Math. Sci. 8 (2013) 41. https://www.m-hikari.com/ijcms/ijcms-2013/1-4-2013/ceruttiIJCMS1-4-2013.pdf.

M. Shakeel, Q. M. Ul-Hassan, J. Ahmad & T. Naqvi, “Exact solutions of the time fractional BBM-Burger equation by novel (G’/G)-expansion method”, Advances in Mathematical Physics (2014) 181594. https://doi.org/10.1155/2014/181594.

W. K. Zahra, “Trigonometric B-spline collocation method for solving PHI-four and Allen-Cahn equations”, Mediterr J. Math. 14 (2017) 122. https://doi.org/10.1007/s00009-017-0916-8.

M. Zellal & K. Belghaba, “Applications of homotopy perturbation transform method for solving Newell Whitehead-Segel equation”, Gen. Lett. Math. 3 (2017) 35. https://doi.org/10.31559/glm2016.3.1.4.

S. T. Mohyud-Din & M. A. Noor, “Modified variational iteration method for solving Fisher’s equations”, J. Appl. Math. Comput. 31 (2009) 295. https://doi.org/10.1007/s12190-008-0212-7.

A. Prakash & M. Kumar, “He’s variational iteration method for the solution of nonlinear Newell Whitehead-Segel equation”, J. Appl. Anal. Comput. 6 (2016) 738. https://doi.org/10.11948/2016048.

Published

2024-05-31

How to Cite

Approximate solution of time-fractional non-linear parabolic equations arising in Mathematical Physics. (2024). African Scientific Reports, 3(2), 176. https://doi.org/10.46481/asr.2024.3.2.176

Issue

Volume 3, Issue 2, August 2024

Section

MATHEMATICAL SCIENCES SECTION
Copyright & Licensing

Copyright (c) 2024 K. Issa, R. A. Bello, M. H. Sulaiman

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Approximate solution of time-fractional non-linear parabolic equations arising in Mathematical Physics. (2024). African Scientific Reports, 3(2), 176. https://doi.org/10.46481/asr.2024.3.2.176